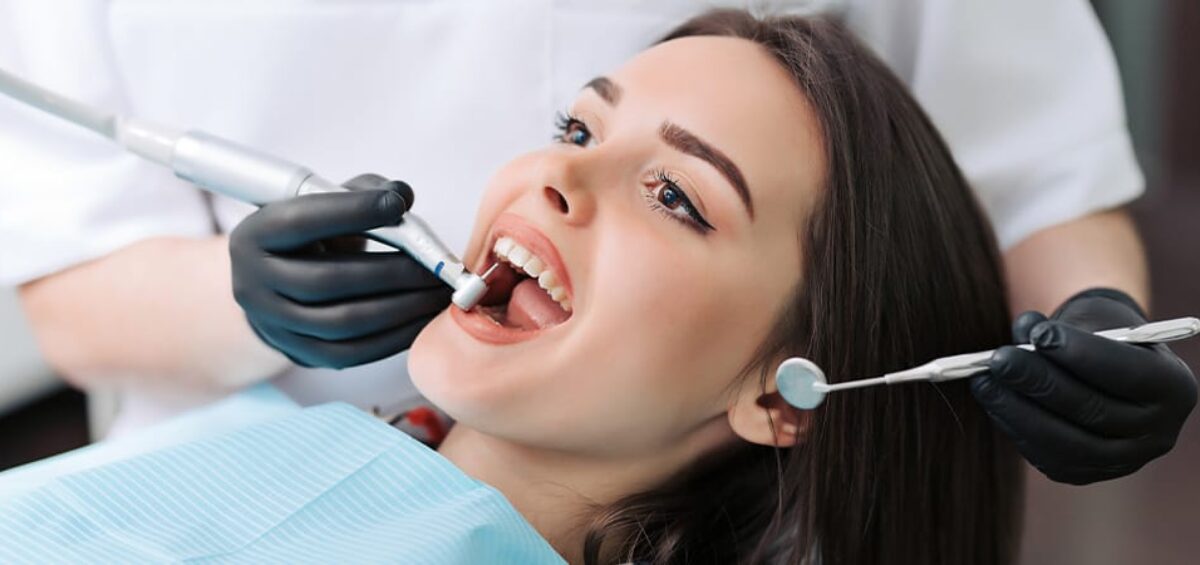
Calculus bridge, also known as tartar bridge, is not a term used in structural engineering or mathematics related to bridges. But it is a dental issue.
Calculus stands as a cornerstone in the realm of engineering, especially in the domain of bridge design and analysis. From understanding the intricate forces at play to optimizing structural elements, calculus plays a vital role in every step of the process. Let’s delve into the world where mathematics meets engineering to create awe-inspiring structures – the world of calculus bridges.
The Basics of Calculus
What is Calculus?
Calculus is a branch of mathematics that deals with the study of rates of change and accumulation of quantities. It comprises two primary branches: differential calculus, which concerns instantaneous rates of change and slopes of curves, and integral calculus, which focuses on the accumulation of quantities and the computation of areas under curves.
Importance of Calculus in Engineering
In the field of engineering, calculus serves as the language of change and motion. It provides engineers with tools to analyze complex systems and predict behaviors, making it indispensable in various disciplines, including physics, mechanics, and structural engineering.
Application of Calculus in Bridge Design
Bridges, as vital components of infrastructure, demand meticulous design and analysis to ensure safety and efficiency. Calculus plays a crucial role in achieving these objectives by enabling engineers to:
Calculating Forces and Stresses
Differential calculus aids in determining the distribution of forces and stresses within bridge components, allowing engineers to identify critical areas prone to structural failure.
Determining Optimal Designs
By employing optimization techniques from calculus, engineers can find the most efficient designs that minimize material usage while meeting structural requirements, leading to cost-effective and sustainable bridge solutions.
Differential Calculus in Bridge Engineering
Differential calculus provides tools to analyze the rate of change in structural elements, facilitating:
Rate of Change in Structural Elements
Engineers utilize derivatives to understand how bridge components deform under various loads, enabling them to design structures that withstand external forces effectively.
Optimization of Bridge Components
Optimization algorithms based on derivatives help engineers refine the dimensions and shapes of bridge elements to enhance performance and longevity.
Integral Calculus in Bridge Engineering
Integral calculus plays a pivotal role in analyzing the accumulation of forces and loads, allowing engineers to:
Area under Curves for Load Distribution
Integrals are employed to calculate the total load exerted on bridge components by considering the area under load distribution curves, ensuring accurate assessment of structural stability.
Calculating Total Forces
By integrating force functions over specific intervals, engineers can determine the cumulative effects of dynamic loads on bridge structures, aiding in the design of robust and resilient bridges.
Calculus Techniques for Bridge Analysis
In addition to basic calculus principles, advanced techniques are utilized in bridge analysis, including:
Differential Equations for Dynamic Bridge Behavior
Differential equations model the dynamic behavior of bridges subjected to varying loads and environmental conditions, allowing engineers to predict responses and ensure structural integrity.
Numerical Methods in Bridge Analysis
Numerical methods, such as finite element analysis and computational fluid dynamics, employ calculus principles to simulate complex interactions between bridge components and external forces, providing insights into performance under real-world conditions.
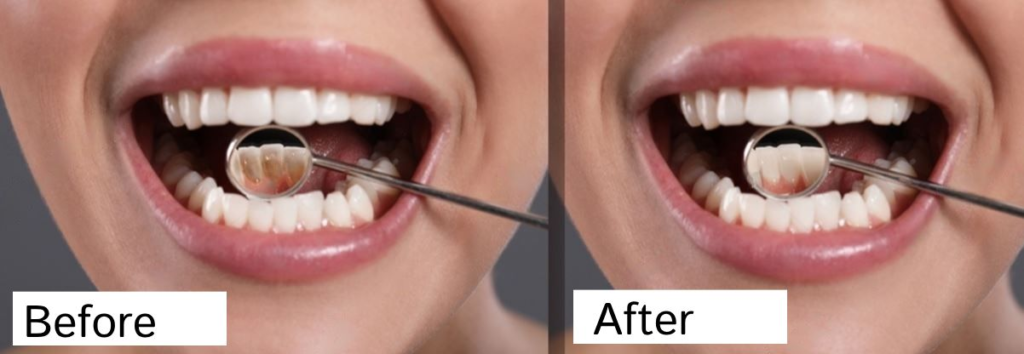
Case Studies: Real-World Applications
Examining real-world examples showcases the practical applications of calculus in bridge engineering, including:
Famous Bridges and Their Calculus Applications
Case studies of iconic bridges like the Golden Gate Bridge and the Millau Viaduct highlight how calculus principles have been instrumental in their design, construction, and ongoing maintenance.
Future Trends: Advancements in Calculus and Bridge Engineering
As technology advances, so too do the tools and techniques available to bridge engineers. Future trends in calculus and bridge engineering include:
- Integration of artificial intelligence and machine learning algorithms for predictive modeling and optimization.
- Development of innovative materials and construction methods to enhance bridge performance and durability.
- Collaboration between mathematicians, engineers, and policymakers to address evolving challenges in infrastructure development and sustainability.
Conclusion
Calculus serves as the mathematical backbone of bridge engineering, enabling engineers to design, analyze, and optimize structures with precision and efficiency. By harnessing the power of calculus, engineers can continue to push the boundaries of bridge design, creating safer, more sustainable infrastructure for generations to come.
FAQs (Frequently Asked Questions)
- How does calculus help in determining the strength of bridge materials?
- Calculus allows engineers to analyze stress distributions and deformations within bridge components, aiding in the selection of materials and designs that meet structural requirements.
- Can calculus predict the lifespan of a bridge?
- While calculus provides tools for analyzing structural behavior, predicting the exact lifespan of a bridge involves considerations beyond mathematical models, such as environmental factors and maintenance practices.
- Are there any limitations to using calculus in bridge design?
- While calculus is a powerful tool, its application in bridge design is not without limitations. Complex interactions between various factors, such as material properties and environmental conditions, can pose challenges in accurately modeling real-world scenarios.
- How do engineers use calculus to address dynamic loads on bridges?
- Differential equations derived from calculus are employed to model the dynamic behavior of bridges subjected to varying loads, allowing engineers to predict responses and ensure structural integrity under dynamic conditions.
- What role does calculus play in bridge maintenance and rehabilitation?
- Calculus principles aid engineers in assessing structural deterioration, identifying critical areas for repair or reinforcement, and optimizing maintenance strategies to prolong the lifespan of bridges.